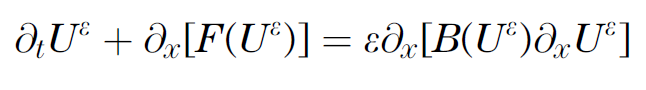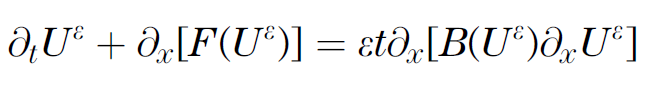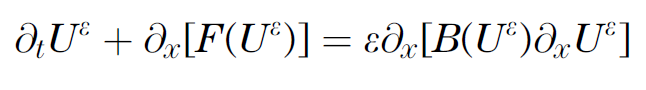
Cleopatra Christoforou and Laura V. Spinolo
On the physical and the self-similar viscous approximation of a boundary Riemann problem
Pages: 41-54
Received: 14 October 2010
Accepted: 24 January 2011
Mathematics Subject Classification (2010): 35L65.
Keywords:Boundary Riemann problem, viscous approximation, self-similar viscous approximation,
boundary layer, characteristic boundary.
Abstract:
We deal with the viscous approximation of a system of conservation laws in one space dimension and
we focus on initial-boundary value problems. It is known that, in general, different viscous approximation
provide different limits because of boundary layer phenomena.
We focus on Riemann-type data and we discuss
a uniqueness criterion for distributional solutions which applies to both the non characteristic and the
boundary characteristic case. As an application, one gets that the limits of the physical viscous
approximation